Warning: jsMath requires JavaScript to process the mathematics on this page.
If your browser supports JavaScript, be sure it is enabled.

2000 II 2

From QED

< PlasmaWiki | Generals(Link to this page as [[PlasmaWiki/Generals/2000 II 2]])

Jump to: navigation, search

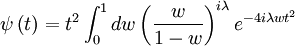
$\psi\left(t\right)=t^{2}\int_{0}^{1}dw\left(\frac{w}{1-w}\right)^{i\lambda}e^{-4i\lambda wt^{2}}$

Writing this as:

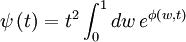
$\psi\left(t\right)=t^{2}\int_{0}^{1}dw\, e^{\phi\left(w,t\right)}$

With:

$\phi\left(w,t\right)=i\lambda\left[\ln\left(w\right)-\ln\left(1-w\right)-4wt^{2}\right]$

Setting the derivative to zero to find saddles:

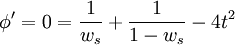
$\phi^{\prime}=0=\frac{1}{w_{s}}+\frac{1}{1-w_{s}}-4t^{2}$

Rearranging:

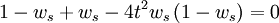
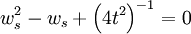
$1-w_{s}+w_{s}-4t^{2}w_{s}\left(1-w_{s}\right)=0$ $w_{s}^{2}-w_{s}+\left(4t^{2}\right)^{-1}=0$

Solving for $w s$ :

$w_{s}=\frac{1\pm\sqrt{1-4\cdot\left(4t^{2}\right)^{-1}}}{2}=\frac{1}{2}\pm\frac{1}{2}\sqrt{1-t^{-2}}$

Which we will now call 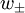$w_{\pm}$ . Looking at the second derivative:

$\phi^{\prime\prime}\left(w\right)=i\lambda\left[-\frac{1}{w^{2}}+\frac{1}{\left(1-w\right)^{2}}\right]$

So that:

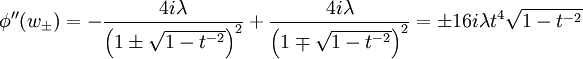
$\phi^{\prime\prime}(w_{\pm})=-\frac{4i\lambda}{\left(1\pm\sqrt{1-t^{-2}}\right)^{2}}+\frac{4i\lambda}{\left(1\mp\sqrt{1-t^{-2}}\right)^{2}}= \pm16i\lambda t^{4}\sqrt{1-t^{-2}}$

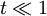For $t\ll1$ , the saddle points are off the axis. Taylor expanding:

$\phi^{\prime\prime}(w_{\pm})=\mp\lambda t^{4}\sqrt{t^{-2}-1}\approx \mp 8\lambda t^{3}$

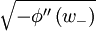So the direction $\sqrt{-\phi^{\prime\prime}\left(w_{+}\right)}$ is along the real axis and the direction $\sqrt{-\phi^{\prime\prime}\left(w_{-}\right)}$ is purely imaginary and cannot be used. We then get from the saddle at $w +$ :

$\psi\left(t\right)=\frac{t^{2}\sqrt{2\pi}}{\sqrt{-\phi^{\prime\prime}\left(w_{+}\right)}}e^{\phi\left(w_{+}\right)}$

Expressing 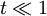$t\ll1$ :

$\phi\left(w_{+},t\right)=i\lambda\left[\ln\left(\frac{1+\sqrt{1-t^{-2}}}{1-\sqrt{1-t^{-2}}}\right)-4\left(1+\sqrt{1-t^{-2}}\right)t^{2}\right]\approx i\lambda\left[\ln\left(-1\right)-4it\right]=4\lambda t-\lambda\pi$

Putting this in:

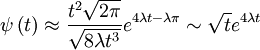
$\psi\left(t\right)\approx\frac{t^{2}\sqrt{2\pi}}{\sqrt{8\lambda t^{3}}}e^{4\lambda t-\lambda\pi}\sim\sqrt{t}e^{4\lambda t}$

For $t\gg1$ , the saddle points are on the real axis. Finding the second derivative:

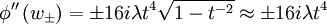
$\phi^{\prime\prime}\left(w_{\pm}\right)=\pm16i\lambda t^{4}\sqrt{1-t^{-2}}\approx\pm16i\lambda t^{4}$

So the direction of integration is 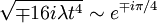$\sqrt{\mp16i\lambda t^{4}}\sim e^{\mp i\pi/4}$ .

We integrate through both saddles. Evaluating $φ$ at the first saddle, with 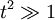$t^{2}\gg1$ :

So this is just a phase factor. At the other saddle:

$\phi\left(w_{-},t\right)=i\lambda\left[\ln\left(\frac{1-\sqrt{1-t^{-2}}}{1+\sqrt{1-t^{-2}}}\right)-4\left(1+\sqrt{1-t^{-2}}\right)t^{2}\right]\approx i\lambda\left[\ln\left(\frac{t^{-2}/2}{2}\right)-8t^{2}\right]=-2i\lambda\ln\left(t/2\right)+8i\lambda t^{2}$

So this is another phase factor. Then the saddles contributions are:

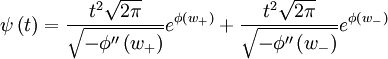
$\psi\left(t\right)=\frac{t^{2}\sqrt{2\pi}}{\sqrt{-\phi^{\prime\prime}\left(w_{+}\right)}}e^{\phi\left(w_{+}\right)}+\frac{t^{2}\sqrt{2\pi}}{\sqrt{-\phi^{\prime\prime}\left(w_{-}\right)}}e^{\phi\left(w_{-}\right)}$

Plugging in:

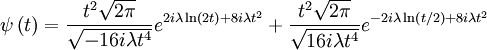
$\psi\left(t\right)=\frac{t^{2}\sqrt{2\pi}}{\sqrt{-16i\lambda t^{4}}}e^{2i\lambda\ln\left(2t\right)+8i\lambda t^{2}}+\frac{t^{2}\sqrt{2\pi}}{\sqrt{16i\lambda t^{4}}}e^{-2i\lambda\ln\left(t/2\right)+8i\lambda t^{2}}$

Which simplifies to:

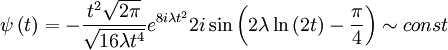
$\psi\left(t\right)=-\frac{t^{2}\sqrt{2\pi}}{\sqrt{16\lambda t^{4}}}e^{8i\lambda t^{2}}2i\sin\left(2\lambda\ln\left(2t\right)-\frac{\pi}{4}\right)\sim const$

This page was recovered in October 2009 from the Plasmagicians page on Generals_2000_II_2 dated 02:50, 29 April 2007.

Retrieved from "https://qed.princeton.edu/main/PlasmaWiki/Generals/2000_II_2"

Category: Plasma Physics Generals