Mean free path

From QED

< PlasmaWiki(Link to this page as [[PlasmaWiki/Mean free path]])
Jump to: navigation, search

The mean free path is generally the distance a particle can travel before it executes a 90 degree collision. This is generally the thermal velocity over the collision frequency :

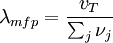
\lambda_{mfp}=\frac{v_T}{\sum_j\nu_j}

A first approximation would be to use the distance of closest approach as the radius, and simply find the first hard scatter:

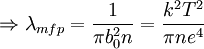
\pi b_0^2 n\lambda_{mfp} = 1 \Rightarrow \lambda_{mfp}=\frac{1}{\pi b_0^2 n}=\frac{k^2T^2}{\pi n e^4}

Expressing this in more fundamental ways:

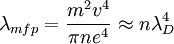
\lambda_{mfp}=\frac{m^2v^4}{\pi n e^4}\approx n\lambda_D^4

However, this is not really correct. Due to the large Debye number , we know that many particles are involved in shielding each other out. This means that many particles are moving at least a little to contribute to the shielding, so that we could think small-angle collisions would play an important role in the mean free path.

The classical mean free path is based on the classical collision frequency . One should find a detailed derivation there, so here we will simply note that the mean free path including small-angle coulomb scattering for electrons is:

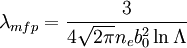
\lambda_{mfp}=\frac{3}{4\sqrt{2\pi} n_e b_0^2 \ln \Lambda}

Where b0 is the distance of closest approach and lnΛ is the coulomb logarithm . For ions note that b0 changes.

This page was recovered in October 2009 from the Plasmagicians page on Mean_free_path dated 01:26, 19 October 2006.

Retrieved from "https://qed.princeton.edu/main/PlasmaWiki/Mean_free_path"
Personal tools