EM M97 2

From QED

< PlasmaWiki | Prelims(Link to this page as [[PlasmaWiki/Prelims/EM M97 2]])
Jump to: navigation, search

Solution 1

PlasmaWiki_Prelims_EM_M97_2.pdf (help ยท info)

Solution 2

An infinite conducting plane (taken here to be the x-y plane) is electrically neutral and carries a uniform surface current 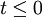t\leq0 and K = K0 for t>0 with K0 equal to a constant.

a. Find the magnitude and direction of the electric and magnetic fields a height z above the plane.

b. Compute the total power radiated per unit area of the x-y plane

The magnetic field is given by:

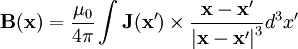
\mathbf{B}(\mathbf{x})=\frac{\mu_{0}}{4\pi}\int\mathbf{J}(\mathbf{x}^{\prime})\times\frac{\mathbf{x}-\mathbf{x}^{\prime}}{\left|\mathbf{x}-\mathbf{x}^{\prime}\right|^{3}}d^{3}x^{\prime}

We have 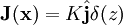\mathbf{J}(\mathbf{x})=K\hat{\mathbf{j}}\delta(z) so taking the cross product and the integral over z :

\mathbf{B}(\mathbf{x})=\frac{\mu_{0}}{4\pi}\int_{z^{\prime}=0}K\hat{\mathbf{i}}\frac{z}{\left|\mathbf{x}-\mathbf{x}^{\prime}\right|^{3}}d^{2}x^{\prime}

Performing this integral:

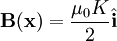
\mathbf{B}(\mathbf{x})=\frac{\mu_{0}K}{2}\hat{\mathbf{i}}

However, this can obviously only travel at the speed of light, so that:

\begin{matrix}\mathbf{B}(|z|&lt;ct) &amp; = &amp; \frac{\mu_0 K}{2}\hat{\mathbf{i}} \\ \mathbf{B}(|z|&gt;ct) &amp; = &amp; 0 \end{matrix}

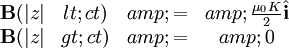
Which gives us an electric field by Faraday's Law:

\nabla\times\mathbf{E}=-\frac{\partial\mathbf{B}}{\partial t}=-\frac{\mu_{0}K}{2}\delta(z+ct)+\frac{\mu_{0}K}{2}\delta(z-ct)

If we think of this as electromagnetic radiation, we wouldn't have E parallel to the direction of travel, and it must be perpendicular to B. Therefore, if we write:

\mathbf{E}=E(\mathbf{x})\hat{\mathbf{y}}

We find:

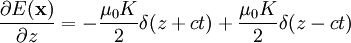
\frac{\partial E(\mathbf{x})}{\partial z}=-\frac{\mu_{0}K}{2}\delta(z+ct)+\frac{\mu_{0}K}{2}\delta(z-ct)
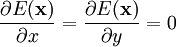
\frac{\partial E(\mathbf{x})}{\partial x}=\frac{\partial E(\mathbf{x})}{\partial y}=0

So that:

\begin{matrix}\mathbf{E}(|z|&lt;ct) &amp; = &amp; -\frac{\mu_0 K}{2}\hat{\mathbf{i}} \\ \mathbf{E}(|z|&gt;ct) &amp; = &amp; 0 \end{matrix}

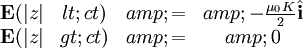
We find the power:

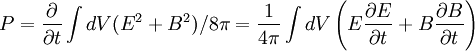
P=\frac{\partial}{\partial t}\int dV(E^{2}+B^{2})/8\pi=\frac{1}{4\pi}\int dV\left(E\frac{\partial E}{\partial t}+B\frac{\partial B}{\partial t}\right)

Per unit area, using symmetry:

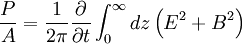
\frac{P}{A}=\frac{1}{2\pi}\frac{\partial}{\partial t}\int_{0}^{\infty}dz\left(E^{2}+B^{2}\right)

Plugging in, using Θ(s) as the heavyside function:

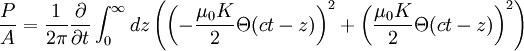
\frac{P}{A}=\frac{1}{2\pi}\frac{\partial}{\partial t}\int_{0}^{\infty}dz\left(\left(-\frac{\mu_{0}K}{2}\Theta(ct-z)\right)^{2}+\left(\frac{\mu_{0}K}{2}\Theta(ct-z)\right)^{2}\right)
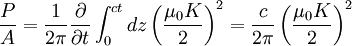
\frac{P}{A}=\frac{1}{2\pi}\frac{\partial}{\partial t}\int_{0}^{ct}dz\left(\frac{\mu_{0}K}{2}\right)^{2}=\frac{c}{2\pi}\left(\frac{\mu_{0}K}{2}\right)^{2}

The power radiated per unit area, as defined on page 347 of Griffiths, is simply the Poynting vector (Given in SI units, with |E| = c |B|):

\frac{\mathbf{P}}{A} = \mathbf{S} = \frac{1}{\mu_{0}} \mathbf{E} \times \mathbf{B} = \frac{\mu_{0} K_{0}^{2} c }{4} \hat{\mathbf{k}}

This page was recovered in October 2009 from the Plasmagicians page on Prelim_M97_EM2 dated 17:34, 20 December 2006.

Retrieved from "https://qed.princeton.edu/main/PlasmaWiki/Prelims/EM_M97_2"
Personal tools